Difference between revisions of "Spring 2020 Assignment 9"

From Course Wiki
Jump to: navigation, search
(→‎Yeast experiment data)
(→‎Yeast experiment data)
Line 140: Line 140:
  
 
==Yeast experiment data==
 
==Yeast experiment data==
−
Unfortunately, we won't be collecting our own data in the lab this semester, but it's still important to get a feel for what the raw data looks like and how the signal manifests itself in those images.  
+
Unfortunately, we won't be collecting our own data in the lab this semester, but it's still important to get a feel for what the raw data look like and how the signal manifests itself in those images.  
  
 
* Download the data file <tt>'fall2019_StudentData_3.mat'</tt> from the [https://www.dropbox.com/sh/j9gdbaoypukdydd/AACP1c3w6JzyzAa--XVpgMV0a?dl=0 Assignment 9 folder] in the course dropbox. The file contains raw data collected by 20.309 students during the Fall 2019 semester.  
 
* Download the data file <tt>'fall2019_StudentData_3.mat'</tt> from the [https://www.dropbox.com/sh/j9gdbaoypukdydd/AACP1c3w6JzyzAa--XVpgMV0a?dl=0 Assignment 9 folder] in the course dropbox. The file contains raw data collected by 20.309 students during the Fall 2019 semester.  
Line 173: Line 173:
  
 
A way to visualize the Hog1 and the nuclear protein simultaneously is to combine the two images into a single color color movie. The code below shows the GFP in cyan and the RFP in red. Areas of high overlap appear white. The code normalizes each frame to optimize the display contrast. It also overlays a square indicator in the upper left corner of the image &mdash; green for low salt and red for high salt.
 
A way to visualize the Hog1 and the nuclear protein simultaneously is to combine the two images into a single color color movie. The code below shows the GFP in cyan and the RFP in red. Areas of high overlap appear white. The code normalizes each frame to optimize the display contrast. It also overlays a square indicator in the upper left corner of the image &mdash; green for low salt and red for high salt.
 +
 +
* ''Tip!'' You can replay the movie in slow motion by changing the replay frame rate! In the movie player window, simply go to the Playback tab, click on Frame Rate... and change the playback frame rate (from 20 fps = 'real time' like the original source movie) to 1 frame per second for instance.
  
 
<pre>
 
<pre>

Revision as of 23:11, 28 April 2020

20.309: Biological Instrumentation and Measurement

ImageBar 774.jpg


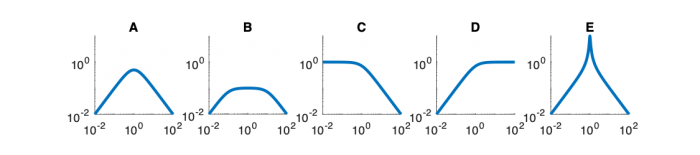
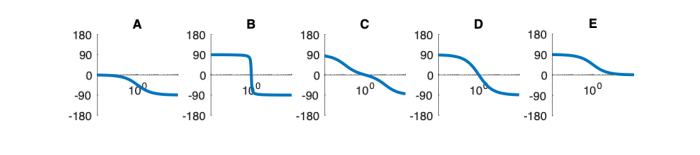
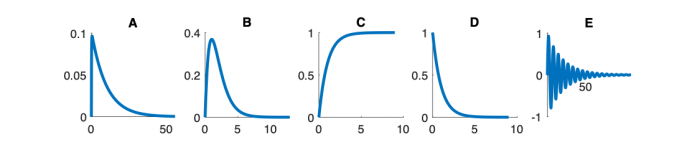
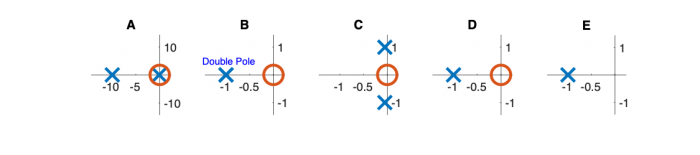
Signals and systems


Pencil.png

Fill out the table below. Match each system function with its Bode magnitude and phase plot, step response, and pole zero diagram. (Write one letter A-E in each box below.) In the row labeled “Description,” write a descriptive name of each system, such as “low-pass filter” or “overdamped second-order system.”


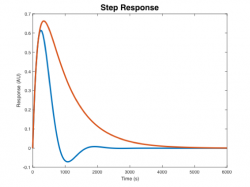
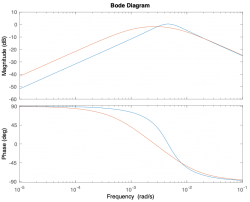
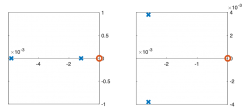
System function $ \frac{1}{s+1} $ $ \frac{s}{s+1} $ $ \frac{s}{s^2+2s+1} $ $ \frac{s}{s^2+0.1s+1} $ $ \frac{1}{s^2+10s+1} $
Magnitude plot
Phase plot
Step response
Pole/zero plot
Description

Magnitude Plots

Transfer function matching magnitude plots.png

Phase Plots

Transfer function matching phase plots.png

Step Response Plots

Transfer function matching step response plots.png

Pole Zero Plots

Transfer function matching pole zero plots.png


Pencil.png
  • Use graphical methods to find the Fourier transform of the half-cosine pulse function x(t) plotted below, which consists of the positive half of a 1 Hz cosine, repeated forever at a rate of 1 Hz.
  • What is the lowest frequency component of x(t), not counting $ \hat{X}(0) $?


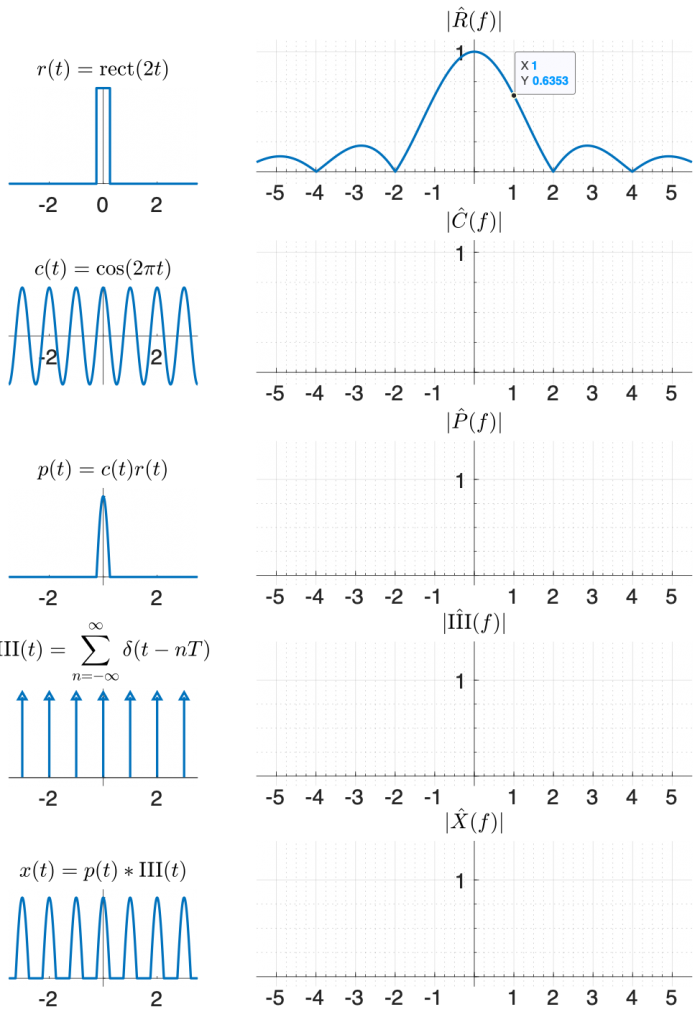
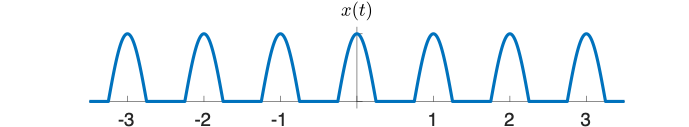Cosine pulse function.png One way to create x(t) using functions that appear on the transform table is:

  1. multiply a cosine by a rectangle, and then
  2. convolve the result with the comb function $ \mathrm{III(}t)=\sum\limits_{n=-∞}^{∞} \delta(t-nT) $.

Use the diagram below to help you find the answer. The left column of shows signals in the time domain, and the right column shows the magnitude of the Fourier transform of each signal. The top right plot is filled in for you, plus a little hint that might help you make an accurate plot.

(The phase of the transforms in this problem is zero at all frequencies, so it is not plotted.)

Cosine pulse transform worksheet.png

Feedback systems


Pencil.png
  • Find the transfer function H ̂(s)=(V ̂_out (s))/(V ̂_in (s)) of the circuit shown below, assuming L = 1 H and R = 1Ω.
  • Plot the poles and zeros of H ̂(s) on a set of axes using x’s for poles and o’s for zeroes.
  • The circuit from Figure 1 is placed in a feedback system, as shown in the block diagram below. The triangle represents an amplifier with gain G that does not depend on frequency. Find the transfer function of the feedback system $ \hat{F}(s)=(\hat{Y}(s))/(\hat{X}(s)). $
  • Plot the poles and zeros of $ \hat{F}(s) $ for G=1,9,and 19. Label the gain value for each point.</math>


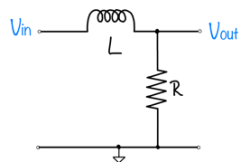
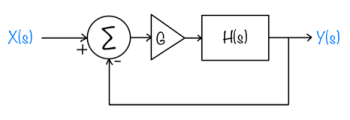
LR Low Pass Filter for S20 Assignment 9.png Feedback System Block Diagram for S20 Assignment 9.png


Pencil.png
  1. Find the transfer function of the circuit below for L = 1 H and R = 1 Ω and C=1 F.
  2. The circuit is placed in the same feedback system shown in the previous question. Plot the poles for gains of 1/4, 3/4, 5/4, 10/4, and 17/4. You may generate your plot by hand or use MATLAB.


LRC circuit for SP20 assignment 9.png

The Frequency Dependence of Osmo-Adaptation in Saccharomyces cerevisiae


Pencil.png

Read The Frequency Dependence of Osmo-Adaptation in Saccharomyces cerevisiae and the supporting information.. This paper will be the focus of exam 2. We will discuss the paper and the supporting information on Thursday and Friday (4/30 and 5/1).

Answer the following questions about The Frequency Dependence of Osmo-Adaptation in S. cerevisiae:

  1. What is the primary mechanism by which S. cerevisiae recovers from hyperosmotic shock?
  2. What mathematical model did Mettetal, et. al. use for Hog1 activation in response to a hyperosmotic shock? Express the model in the following forms
    • transfer function (TF)
    • poles and zeros (ZPK)
    • single differential equation (SDE)
    • coupled differential equations (CDE)
    Express the TF, SDE, and ZPK models in terms of the undamped natural frequency, $ \omega_0 $, damping ratio $ \zeta $, and/or damped natural frequency $ \omega_D $.
  3. What mathematical model did Mettetal, et. al. use to account for nonlinearities in the system?
  4. Plot the frequency response (i.e. make a Bode plot) of the model over a range of $ \omega_0 $ and $ \zeta $ values that includes over damped, critically damped, and under damped.
  5. Find an expression for the step response and plot it over a range of values of $ \omega_0 $ and $ \zeta $. A hand-drawn plot is fine, but you should probably look into MATLAB's step function.
  6. Mettetal, et. al. found that the hyperosmotic shock response of wild-type yeast was (choose one): underdamped, critically damped, or overdamped.
  7. The response of the mutant (low Pbs) yeast was (choose one): underdamped, critically damped, or overdamped.
  8. Which of the step responses below corresponds to Mettetal's model for the wild-type strain and the mutant strain (neglecting the nonlinear element)?
  9. Which of the Bode plots below corresponds to Mettetal's model for the wild-type strain and the mutant strain?
  10. Which of the pole zero diagrams below corresponds to Mettetal's model for the wild-type strain and the mutant strain?
  11. What are two questions that you have about the paper's methodology?

    12. </div>


Mettetal yeast model step response.png Mettetal yeast model Bode plots.png Mettetal yeast model pole zero diagrams.png

Yeast experiment data

Unfortunately, we won't be collecting our own data in the lab this semester, but it's still important to get a feel for what the raw data look like and how the signal manifests itself in those images.

  • Download the data file 'fall2019_StudentData_3.mat' from the Assignment 9 folder in the course dropbox. The file contains raw data collected by 20.309 students during the Fall 2019 semester.
  • Load the file into your MATLAB workspace. You should see a variable called yeastOsmoticShockData, a struct which contains the movie data plus other relevant experimental parameters: 
>> yeastOsmoticShockData

yeastOsmoticShockData = 

  struct with fields:

                         Movie: [544×728×2×32 uint16]
                          Time: [32×2 double]
                    ValveState: [32×1 logical]
        ValveOscillationPeriod: 480
     BlueCameraGainAndExposure: [3 5000000]
    GreenCameraGainAndExposure: [15 5000000]

The engineered cells we used had 2 fluorescent proteins: a Hog1-GFP fusion and a nuclear protein tagged with RFP. Accordingly, the movie data has 2 color planes. The size of the movie's third dimension is 2. (It was 1 for the monochrome images we took in lab earlier this semester.)

  • Color plane 1 is the GFP-Hog1 image, taken with blue illumination and green emission.
  • The second color plane shows the RFP nuclear tag protein, taken with yellow/green illumination and red emission filters.

Use implay to watch each color of the movie: 

implay(double(yeastOsmoticShockData.Movie(:,:,1,:))/4095);
implay(double(yeastOsmoticShockData.Movie(:,:,2,:))/4095);

Can you see how the distribution of Hog1 changes when the cell undergoes osmotic shock?

A way to visualize the Hog1 and the nuclear protein simultaneously is to combine the two images into a single color color movie. The code below shows the GFP in cyan and the RFP in red. Areas of high overlap appear white. The code normalizes each frame to optimize the display contrast. It also overlays a square indicator in the upper left corner of the image — green for low salt and red for high salt.

  • Tip! You can replay the movie in slow motion by changing the replay frame rate! In the movie player window, simply go to the Playback tab, click on Frame Rate... and change the playback frame rate (from 20 fps = 'real time' like the original source movie) to 1 frame per second for instance. 
close all

combinedMovie = zeros( size( yeastOsmoticShockData.Movie ) + [ 0 0 1 0 ] );
normalize = @( x ) ( x - min( x(:) ) ) / range( x(:) );

for ii = 1: size( yeastOsmoticShockData.Movie, 4 )
  gfpImage = normalize( double( yeastOsmoticShockData.Movie(:,:,1,ii) ) );
  gfpImageWithIndicator = gfpImage;
  gfpImageWithIndicator(10:50,10:50) = 1 - yeastOsmoticShockData.ValveState(ii);
  rfpImage = normalize( double( yeastOsmoticShockData.Movie(:,:,2,ii) ) );
  rfpImageWithIndicator = rfpImage;
  rfpImageWithIndicator(10:50,10:50) = yeastOsmoticShockData.ValveState(ii);
  combinedMovie(:,:,:,ii) = cat( 3, rfpImageWithIndicator, gfpImageWithIndicator, gfpImage );
end

implay( combinedMovie )


Pencil.png
  1. Step through the frames of the movie using the implay controls (for either the raw data or the color movie, your choice). Identify a frame where the signal is "high" - in other words, where the Hog1 signal is localized in the nucleus. Turn in the frame number that you've identified and a screen shot of the GFP-Hog1 movie at that frame number.
  2. Repeat for a frame where the signal is "low" - in other words, where the Hog1 signal is uniformly distributed throughout the cell. Turn in the frame number that you've identified and a screen shot of the GFP-Hog1 movie at that frame number.


</div>
Retrieved from "http://engineerbiology.org/w/index.php?title=Spring_2020_Assignment_9&oldid=44209"